Euclidea 2.3 Angle of 30°

We can achieve 3L and 3E in one go, as below:
Don't forget the 2V - one above the line one beneth it.

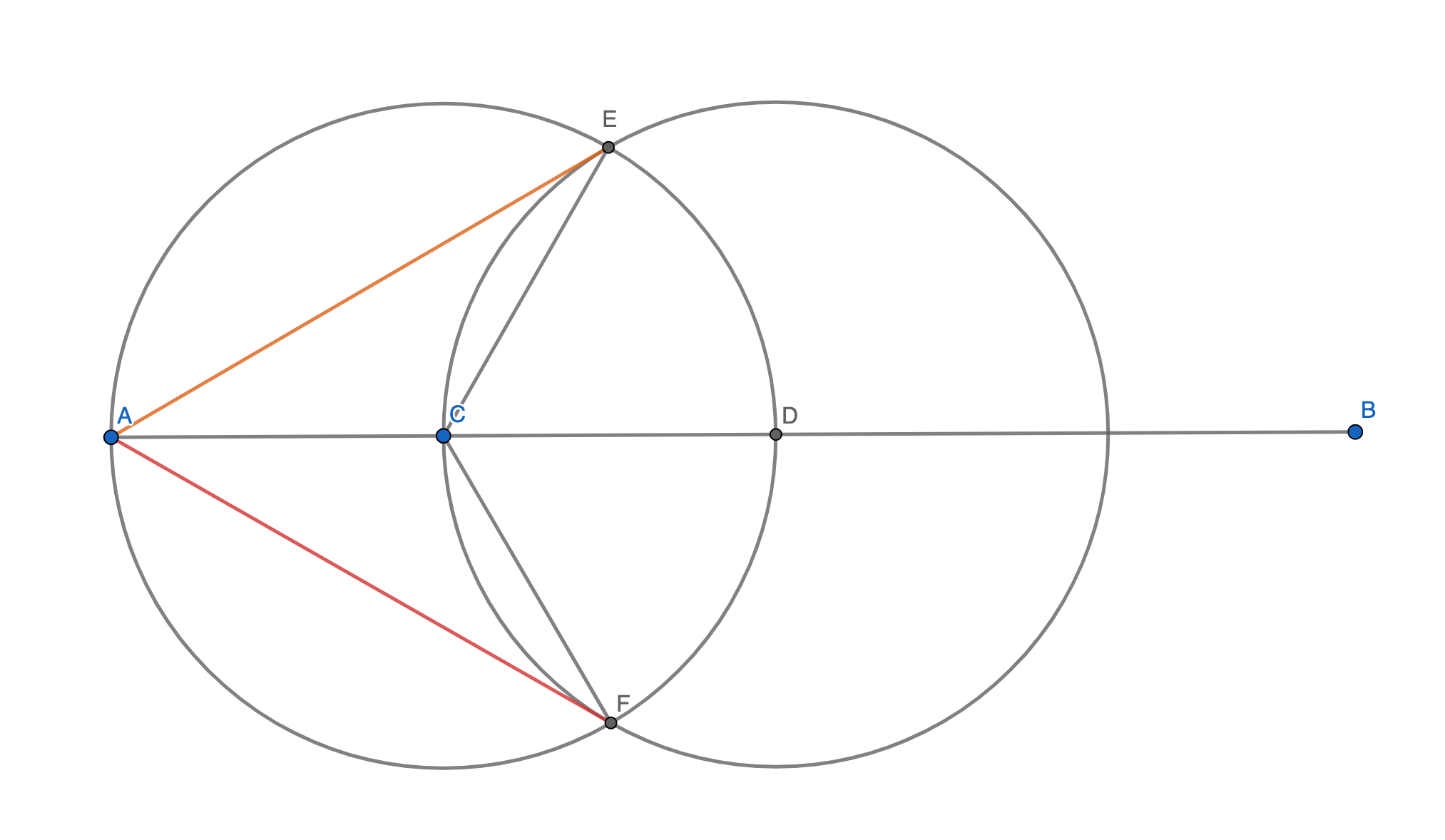
Detailed Steps

• We are given segment \(AB\)
• Random pick a point \(C\), draw circle \( C\) pass \(A\), intersecting \(AB\) at point \(D\)
• Draw circle \(D\) pass \(C\). The 2 circle intersect at point \(E\) and \(F\)
• Connect \(AE\) and \(AF\), they are the 30° we want.

Proof

• \(\triangle CDE\) is equilateral triangle, we have \(\angle EDC\) = 60°
• \(\triangle ADE\) is right triangle, since \(AD\) is diamater of circle.
• Thus, \(\angle DAE\) = 90° - 60° = 30°